[洛谷 2765]魔术球问题

题目链接

https://www.luogu.com.cn/problem/P2765

题解

不管咋样，我们先画一下图找找规律。

如果 \(x\) 能放在 \(y\) 上面，就从 \(y\) 向 \(x\) 连一条边。

比如样例对应的情况是这样的：

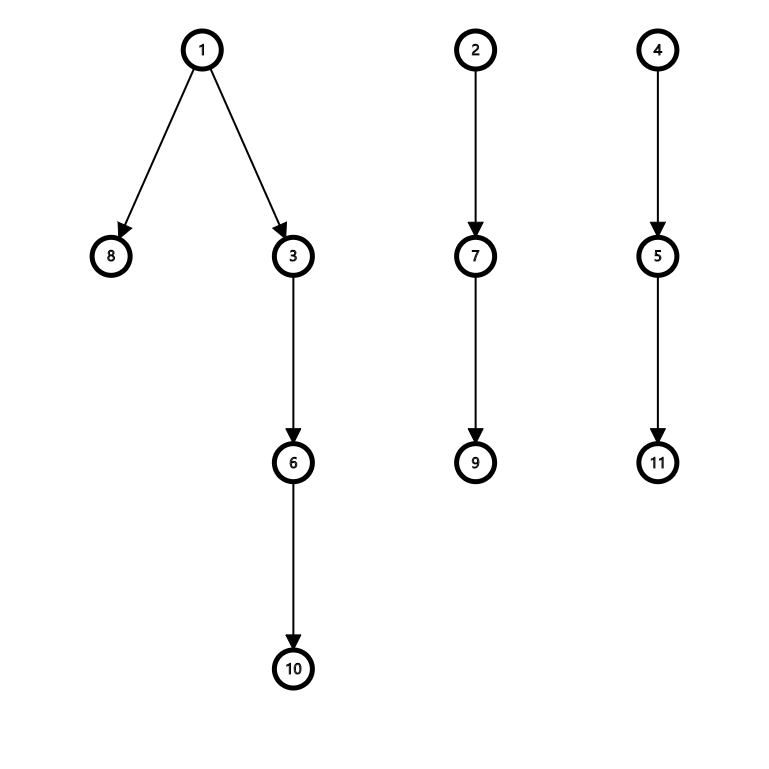
我们的目标是让我们用到的柱子数最少。也就是说，本题的任务是找一个 DAG 的（不重复的）最小路径覆盖。

因此我们可以这样做：我们每次动态加入一个点，并连接满足题意的边。跑 DAG 的最小路径覆盖，当最小路径覆盖数大于 \(n\) 时（也就是说 \(n\) 个柱子放不下了）则停止。

DAG 的最小路径覆盖可以参考 最小路径覆盖问题 一题。

本题中加点和加边是动态进行的，我们只需在上一次的残量网络上跑流就行。

本题还要输出方案。我们只需在残量网络上求出每个点的后继节点即可。

#include <cstdio>
#include <cstring>
#include <algorithm>
#include <queue>
#define INF 0x3f3f3f3f
using namespace std;
struct edge
{
 int v,w,next;
}e[100005];
int s=5e4+1,t=5e4+2,cnt=1;
int head[100005],dep[100005],vis[100005],cur[100005],nxt[100005];
void addedge(int u,int v,int w)
{
 e[++cnt].v=v;
 e[cnt].w=w;
 e[cnt].next=head[u];
 head[u]=cnt;
}
bool bfs()
{
 queue<int> q;
 memset(dep,INF,sizeof(dep));
 memset(vis,0,sizeof(vis));
 memcpy(cur,head,sizeof(head));
 dep[s]=0;
 vis[s]=1;
 q.push(s);
 while(!q.empty())
 {
  int p=q.front();
  q.pop();
  vis[p]=0;
  for(int i=head[p];i;i=e[i].next)
   if(dep[e[i].v]>dep[p]+1&&e[i].w)
   {
    dep[e[i].v]=dep[p]+1;
    if(!vis[e[i].v])
    {
     vis[e[i].v]=1;
     q.push(e[i].v);
    }
   }
 }
 if(dep[t]==INF)return 0;
 return 1;
}
int dfs(int p,int w)
{
 if(p==t)return w;
 int used=0;
 for(int i=cur[p];i;i=e[i].next)
 {
  cur[p]=i;
  if(dep[e[i].v]==dep[p]+1&&e[i].w)
  {
   int flow=dfs(e[i].v,min(w-used,e[i].w));
   if(flow)
   {
    used+=flow;
    e[i].w-=flow;
    e[i^1].w+=flow;
    if(used==w)break;
   }
  }
 }
 return used;
}
bool check(int x)
{
 for(int i=1;i*i<=x;i++)
  if(i*i==x)return true;
 return false;
}
int main()
{
 int n;
 scanf("%d",&n);
 int ans=1,num=0;
 while(1)
 {
  int p=ans*2-1,q=ans*2;
  addedge(s,p,1);
  addedge(p,s,0);
  addedge(q,t,1);
  addedge(t,q,0);
  for(int i=1;i<ans;i++)
   if(check(ans+i))
   {
    addedge(i*2-1,q,1);
    addedge(q,i*2-1,0);
   }
  while(bfs())
   num+=dfs(s,INF);
  if(ans-num>n)
  {
   printf("%d",ans-1);
   for(int u=1;u<ans;u++)
    for(int i=head[u*2-1];i;i=e[i].next)
     if(e[i].v/2<ans&&!e[i].w)nxt[u]=e[i].v/2;
   for(int i=1;i<ans;i++)
    for(int j=i;j&&!vis[j];j=nxt[j])
    {
     if(j==i)puts("");
     printf("%d ",j);
     vis[j]=1;
    }
   return 0;
  }
  ans++;
 }
}